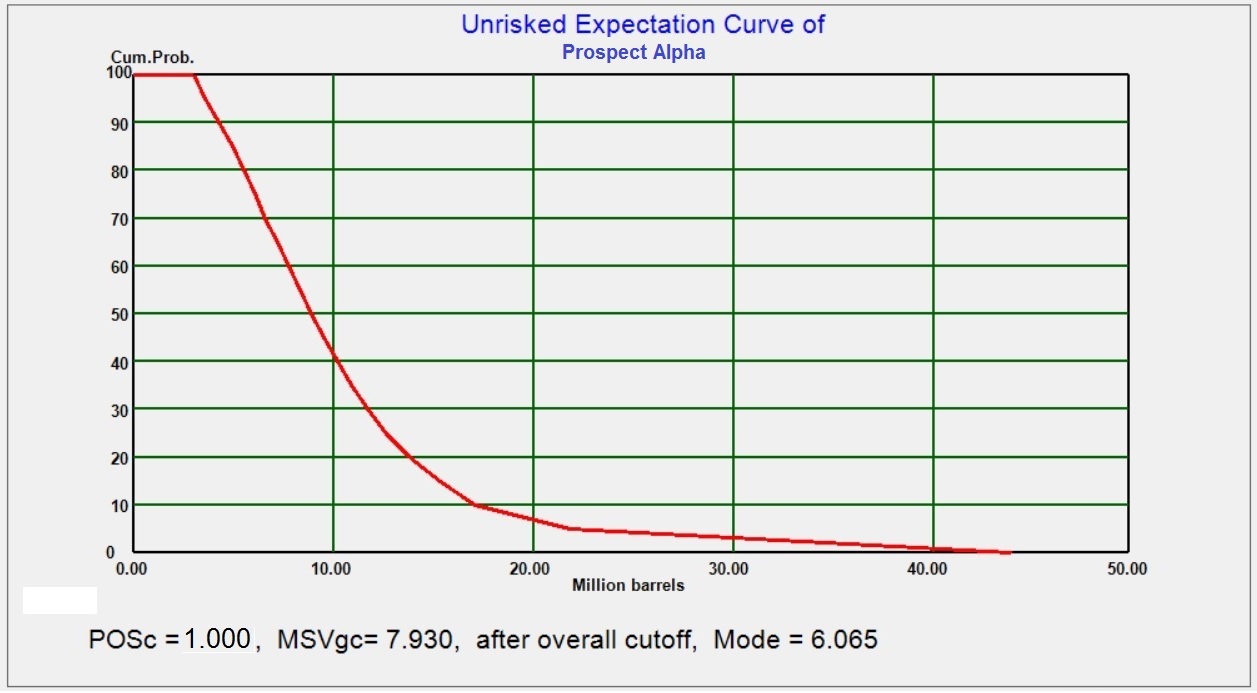
Expectation curve --- Definition
An expectation curve ("EC", sometimes called "Risk Curve", or "Exceedance Curve") is the graphical representation of the distribution of possible outcomes for an uncertain situation, e.g. the volume of HC in a prospect before drilling. It is the complementary distribution function which means that the probabilities given on the plot are the probabilities to exceed a given value, rather than being less than the x-value as used in statistics. Another term for the expectation curve is "Continuous probability exceedance distribution", which is too much of a mouthful. Very often the words -- expectation curve - refer to the statistical distribution as well as to the graph of it. The expectation curve is the standard representation used in resource assessments. The example below could be the EC for the recoverable oil in an undrilled prospect.
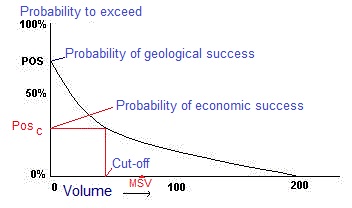
The POS is the Probability Of Success, here about 70%. Conversely, there is a 100 - 70 = 30% chance to find nothing. (Note: the POS is called "COS" or "Chance of Success" by some.) The curve shows about 20% chance to exceed 100 but that a volume of more than 200 is thought to be impossible. But note that the probability of success is a "geological" one which may not realistic in economic terms as the POS depends on your definition of success. See MSV. for a POS after economic cutoff.
Although the above graph shows the whole uncertainty about a prospect, in practice the so-called "unrisked expectation curve" is mainly used. The curve shows the distribution of possible outcomes that exceed the cut-off economic volume. The curve starts at 100% probability on the left axis. The volume in a discovery already made would have a similar curve. From this curve the percentiles are extracted for reporting: the P90, P50 and P10, and for an economic cashflow analysis. At a later stage in the decision making the POSc is taken into account, to include the complete uncertainty.